导数五步法画函数图像10个函数示意图应用举例之一

\n\n

\n\n

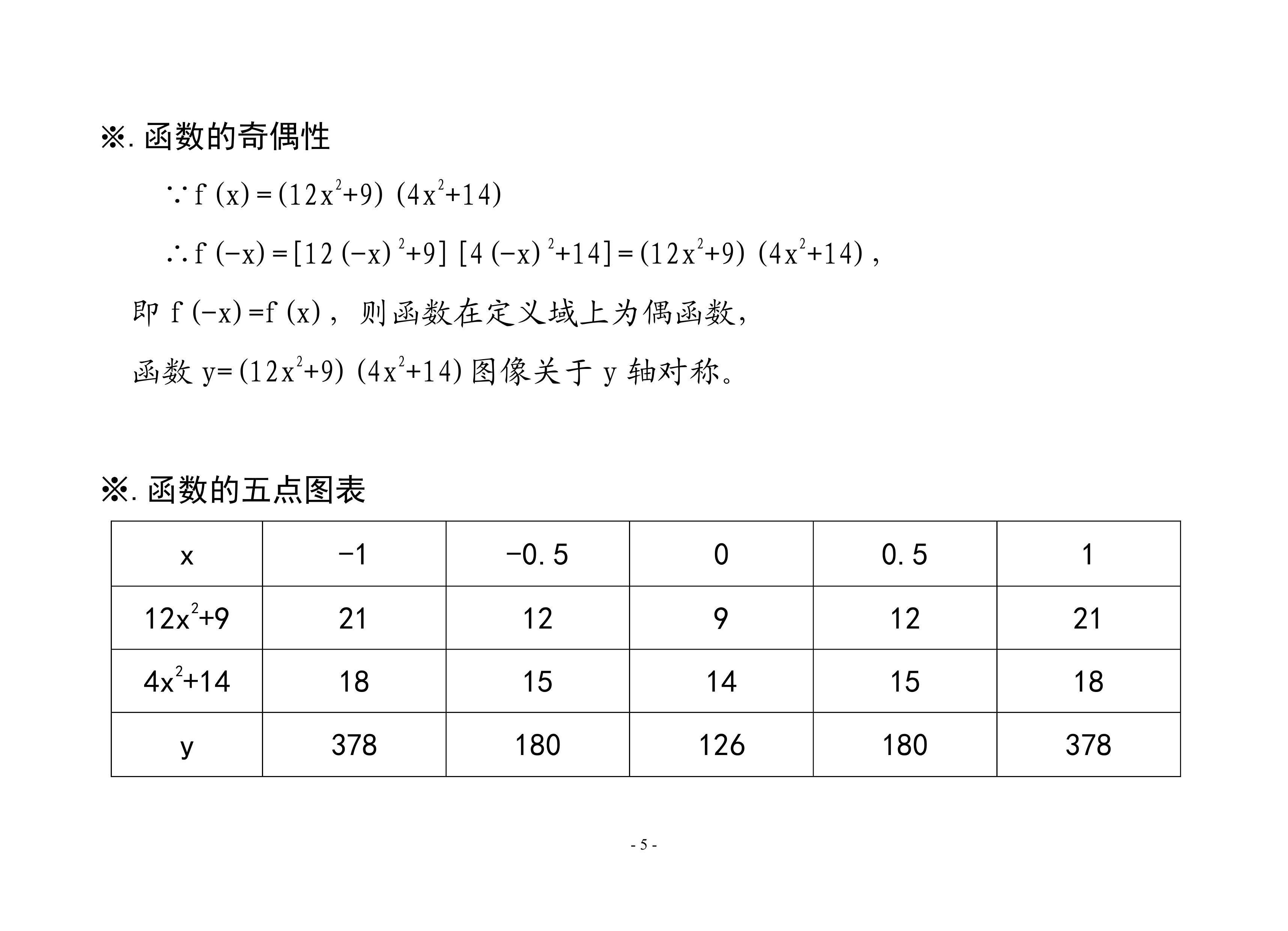
1.函数y=(12x2+9)(4x2+14)的图像示意图：介绍函数的定义域、单调性、凸凹性、极限等性质及五点图表，并通过导数知识计算函数的单调和凸凹区间，简要画出示意图。

\n\n

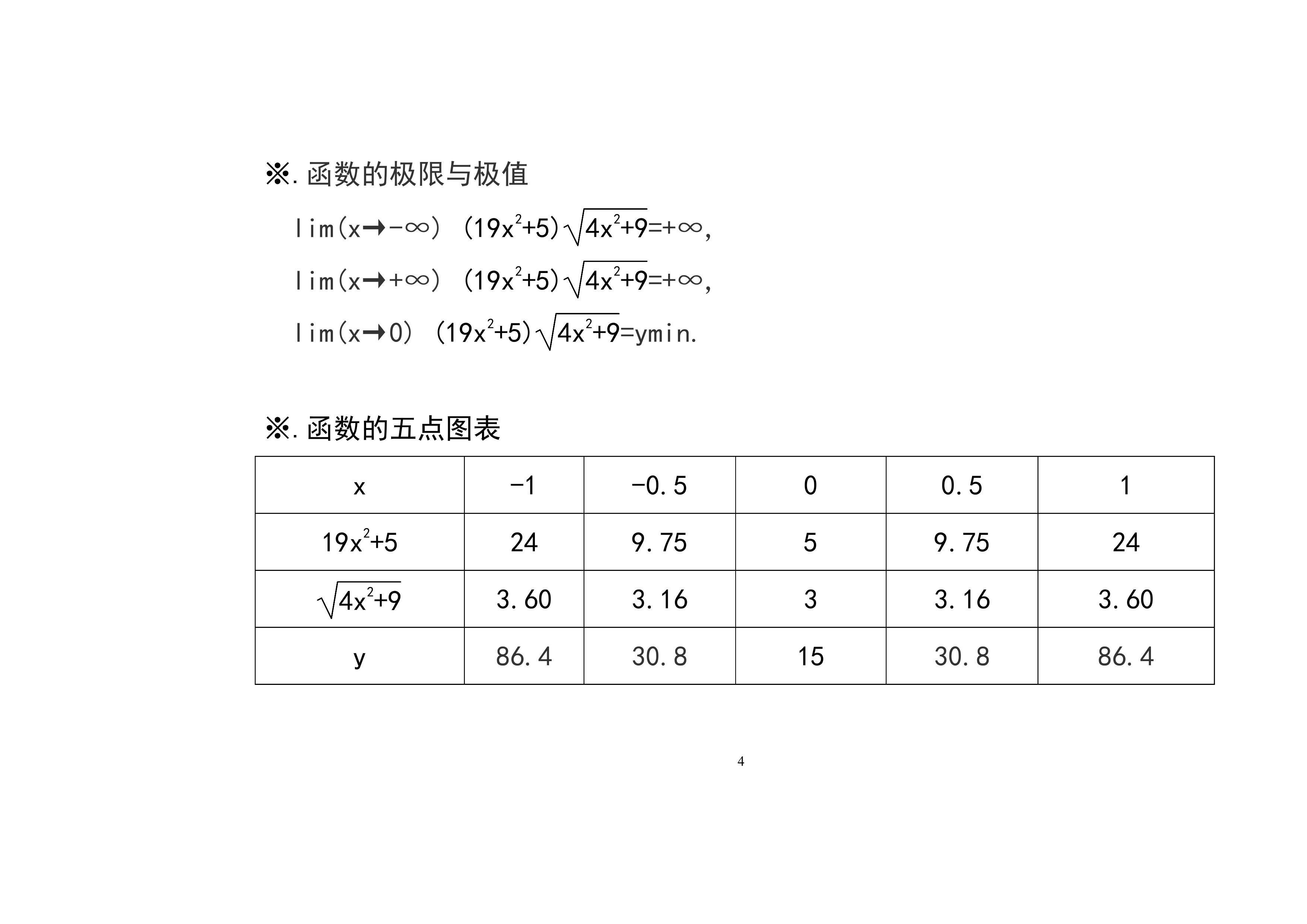
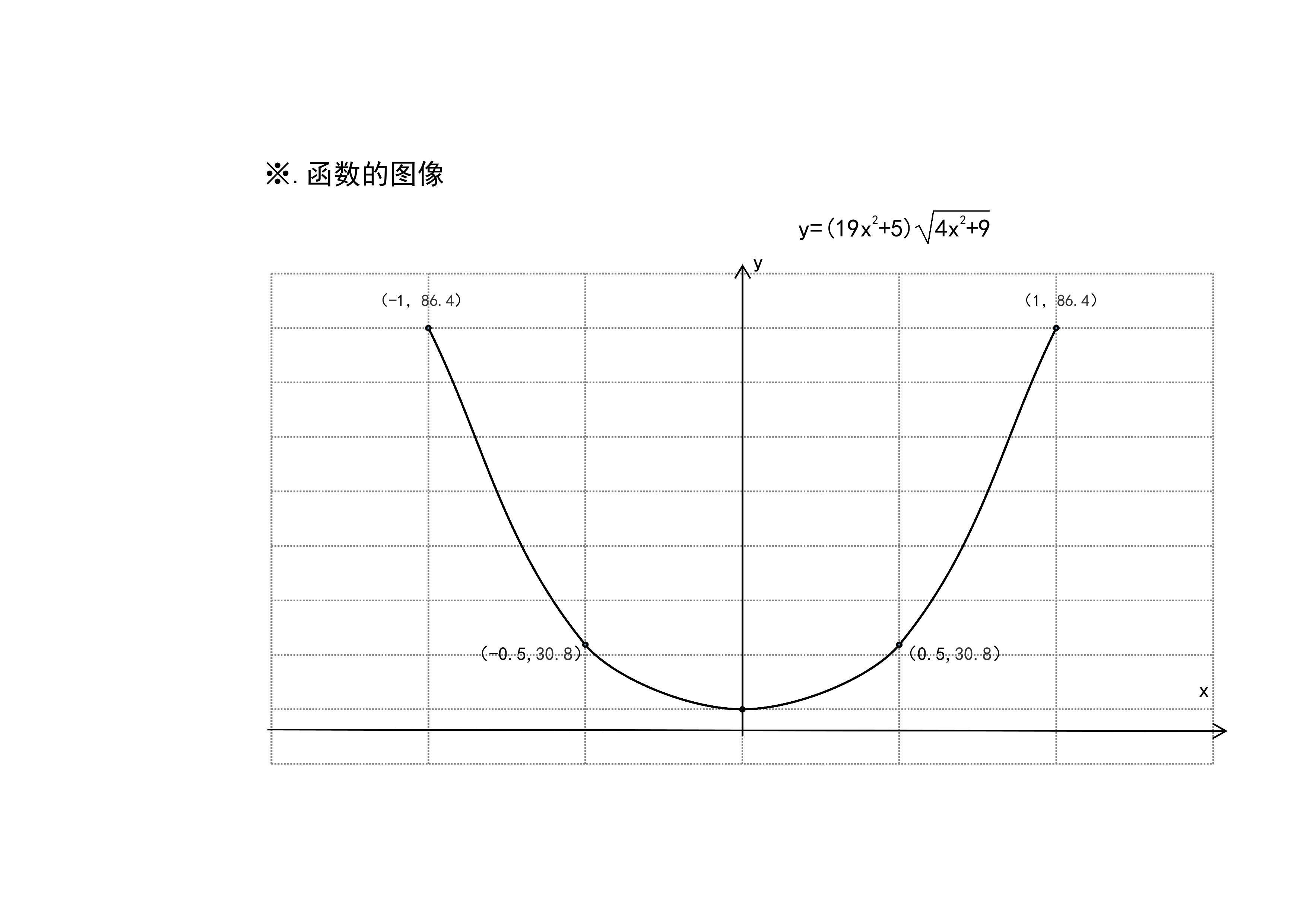
2.函数y=(19x2+5)√(4x2+9)的主要性质及其图像：介绍函数的定义域、单调性、凸凹性、极限等性质，列举函数的五点图表，进一步画出函数的示意图。

\n\n

3.函数y=4√(x+80)^7图像画法及步骤：本文通过函数的定义、单调、凸凹性和极限等性质，介绍函数的主要性质及图像画法步骤。

\n\n

4.曲线x³+y³=2的主要性质及其图像示意图：介绍曲线方程的定义域、单调性、凸凹性等性质，同时用导数的知识求解函数的单调区间和凸凹区间，并简洁画出函数的图像示意图。

\n\n

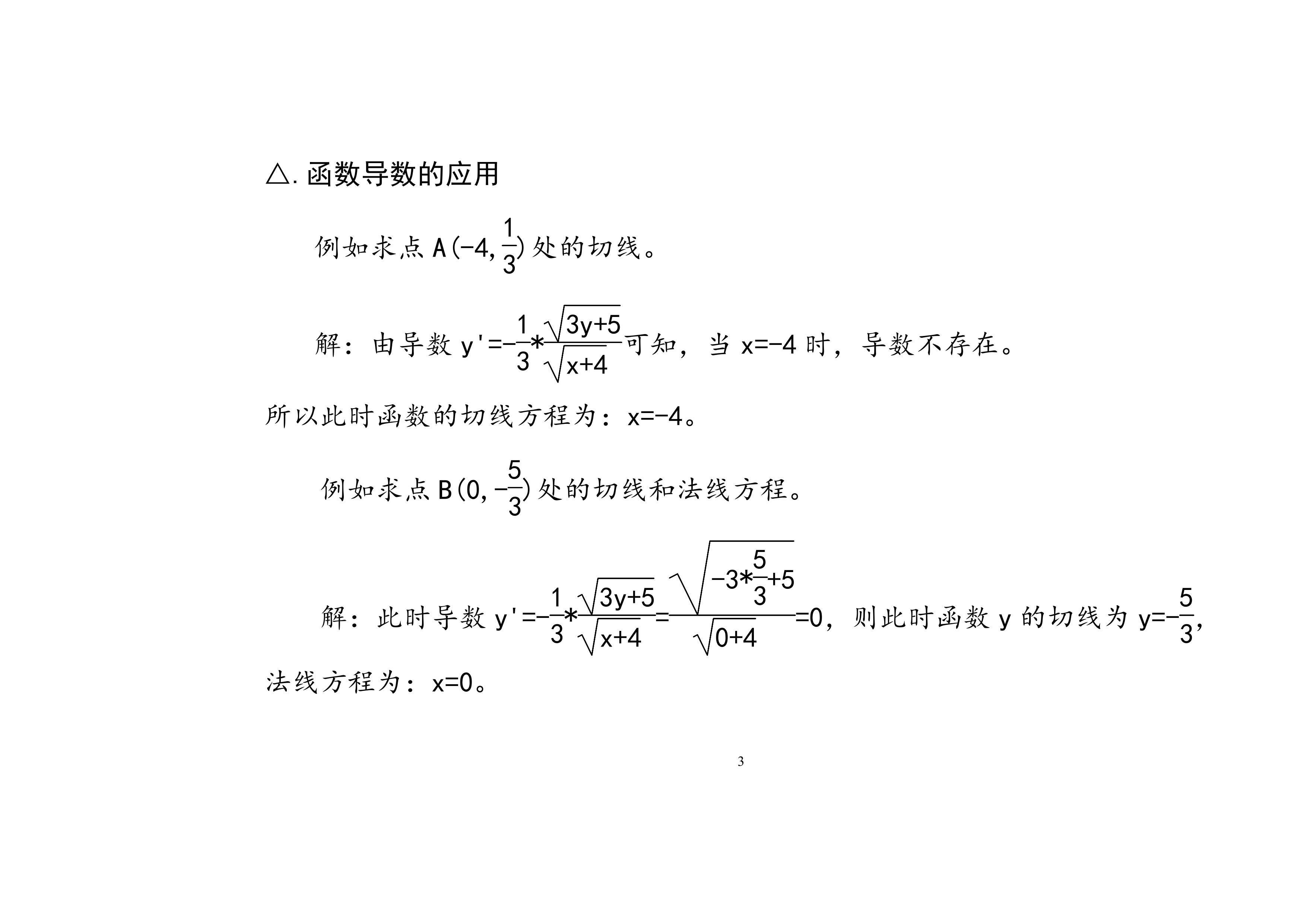
5.√(x+4)+√(3y+5)=2的图像示意图：介绍曲线方程的定义域、单调性、凸凹性及极限等性质，同时用导数简洁画出函数的图像示意图。

\n\n

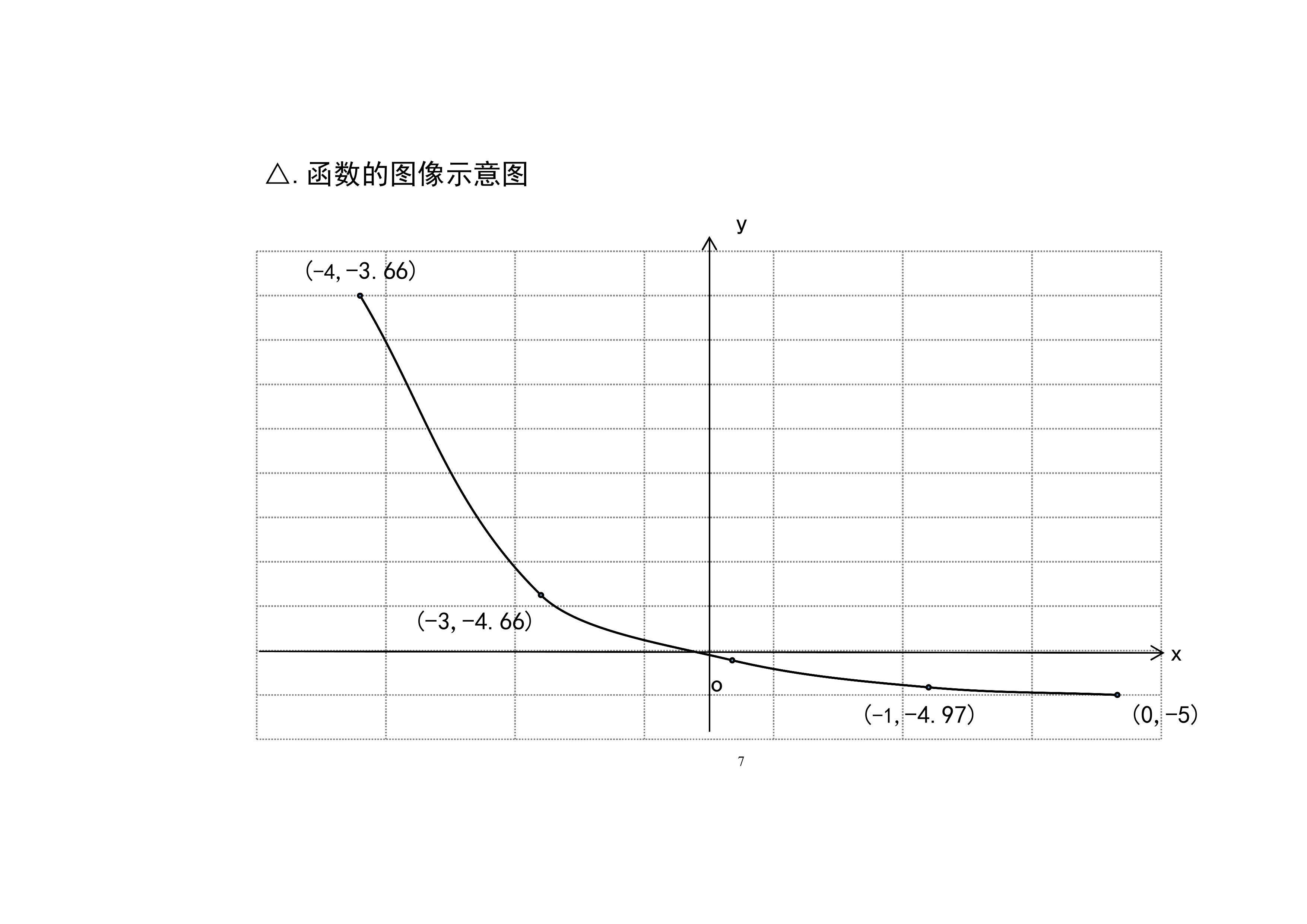
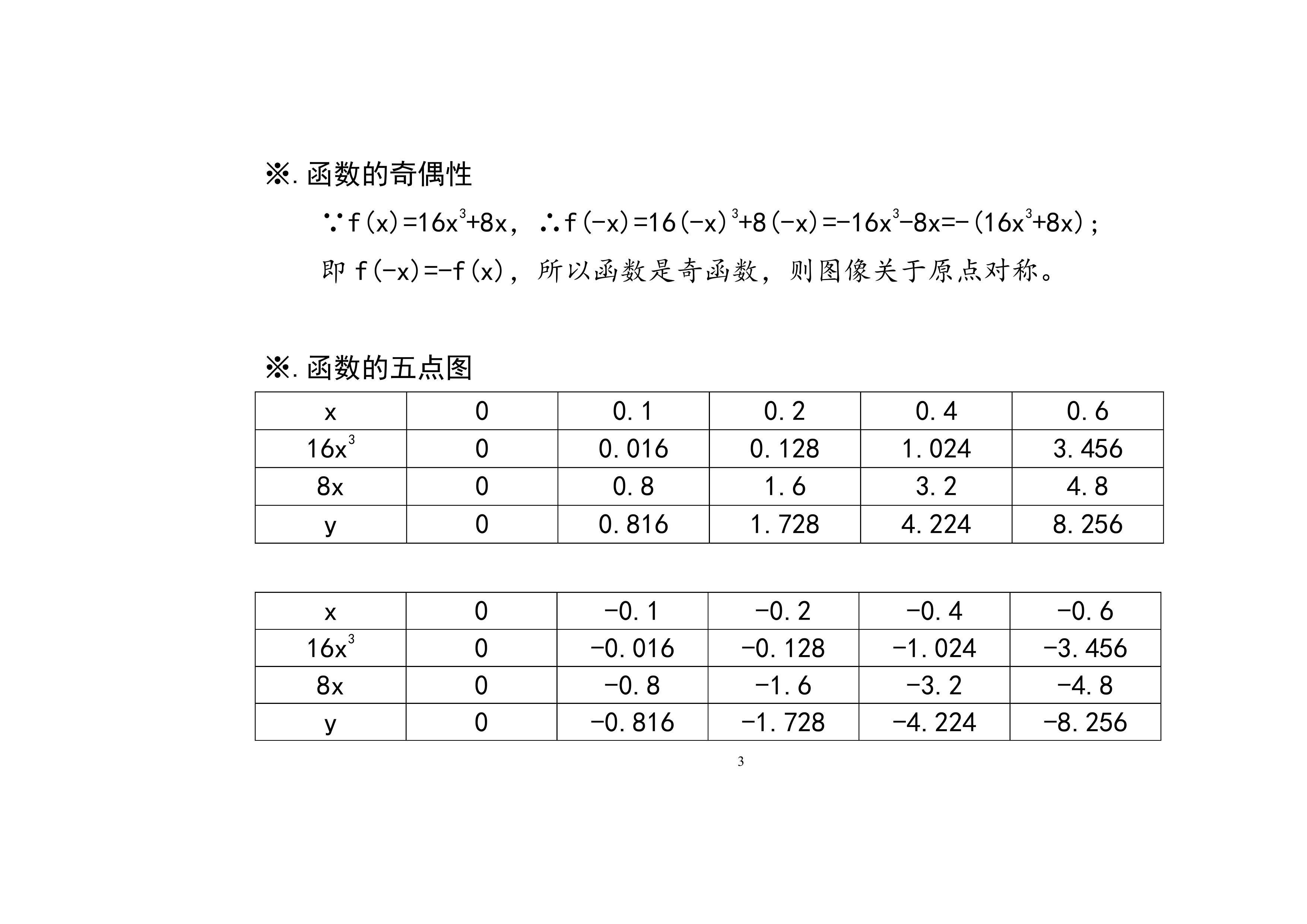
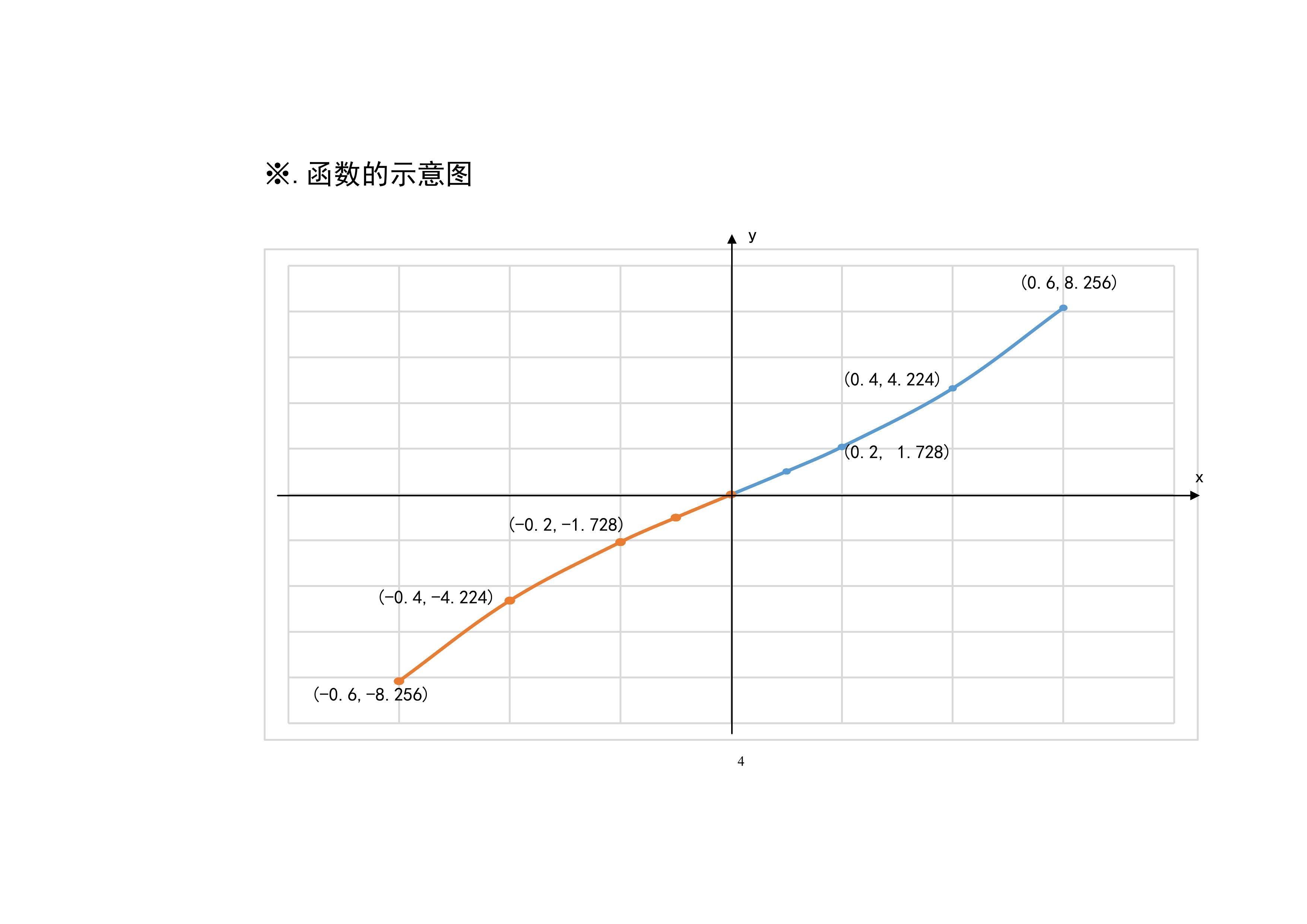
6.函数y=16x3+8x的图像示意图及主要性质：介绍函数的定义域、单调性、凸凹性、极限等性质，列举函数的五点图表，进一步画出函数的示意图。

\n\n

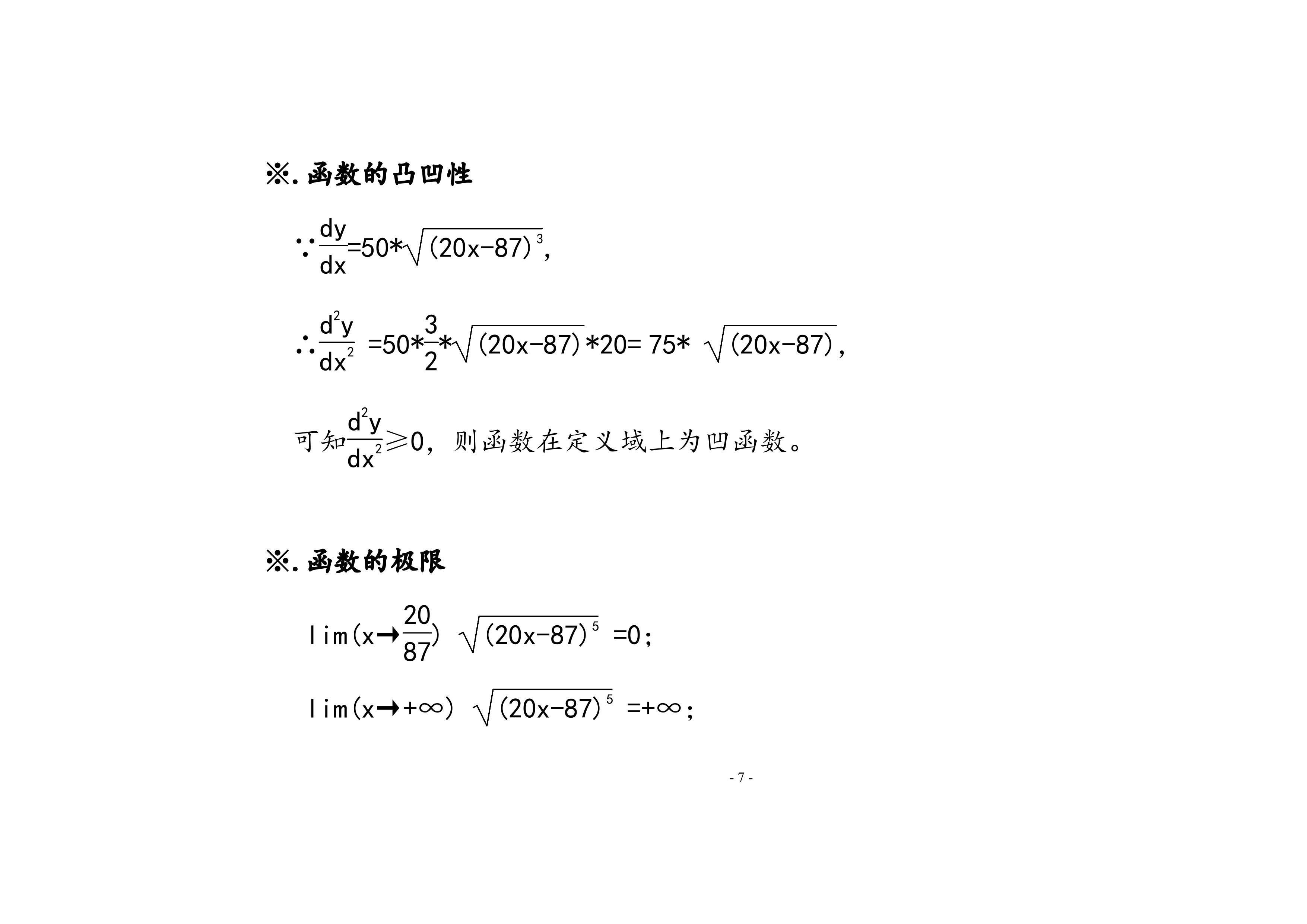
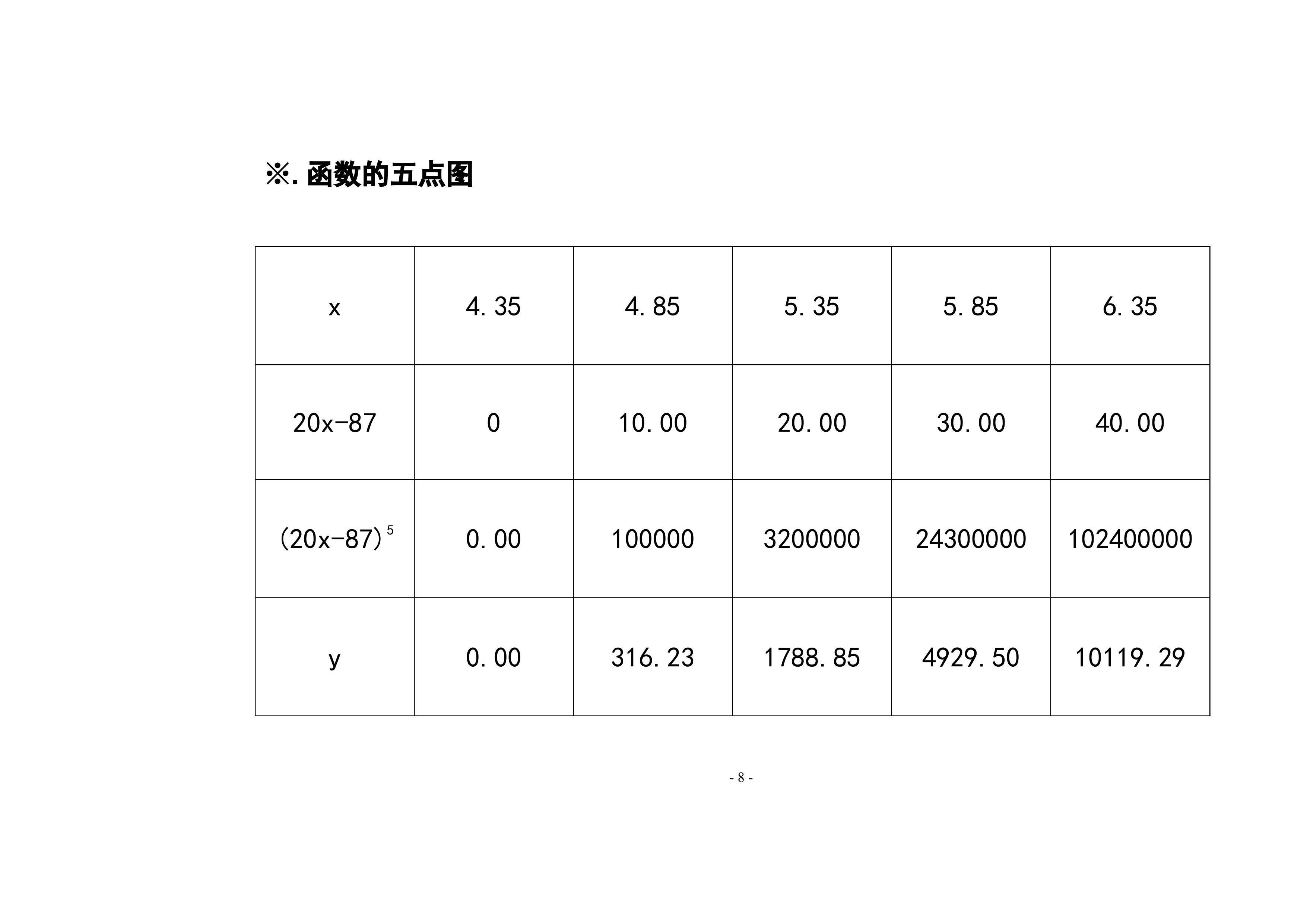
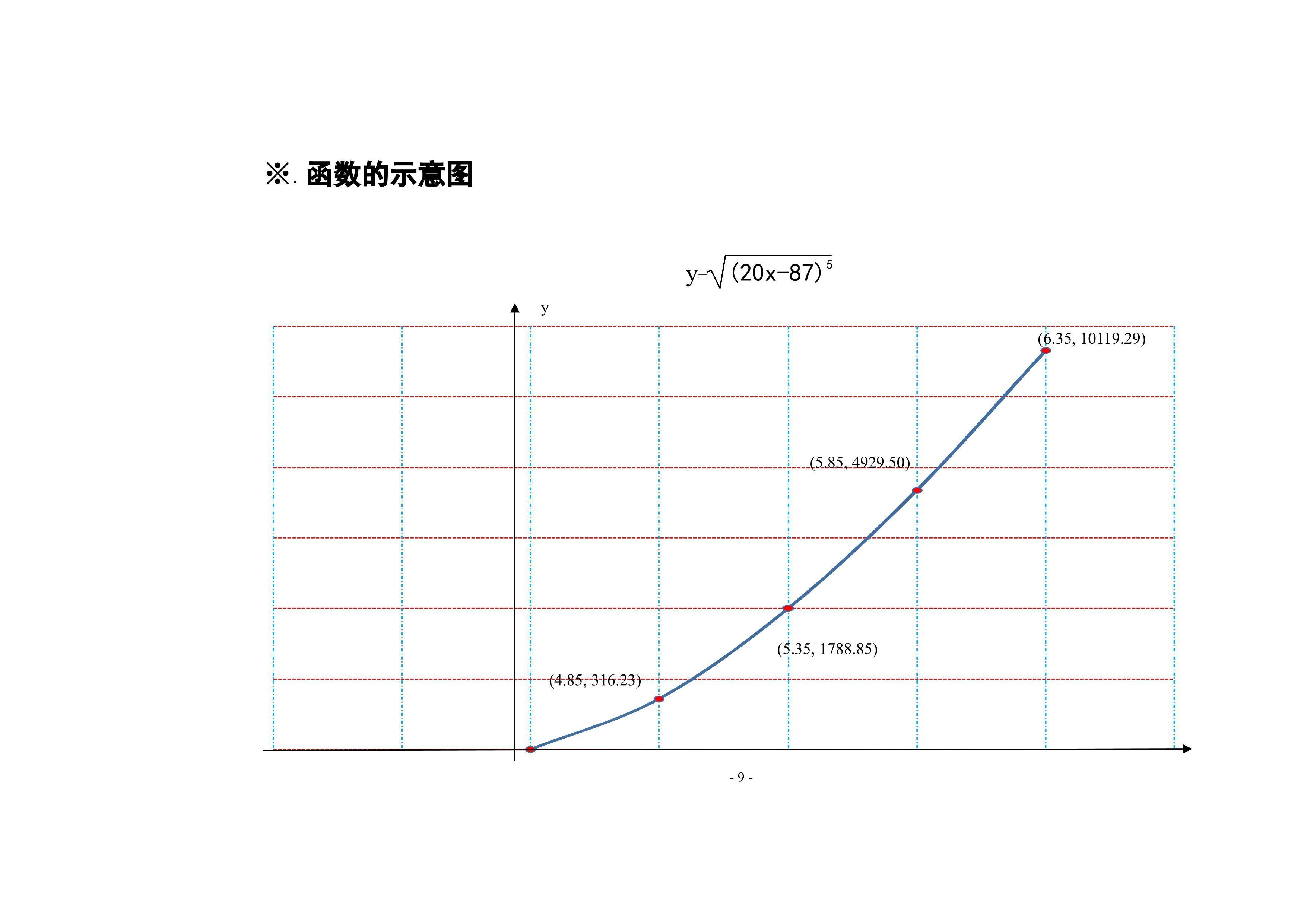
7.函数y=√(20x-87)^5图像画法及步骤：通过函数的定义、单调、凸凹和极限等性质， 并通过导数知识，介绍函数的主要性质及图像示意图画法步骤。

\n\n

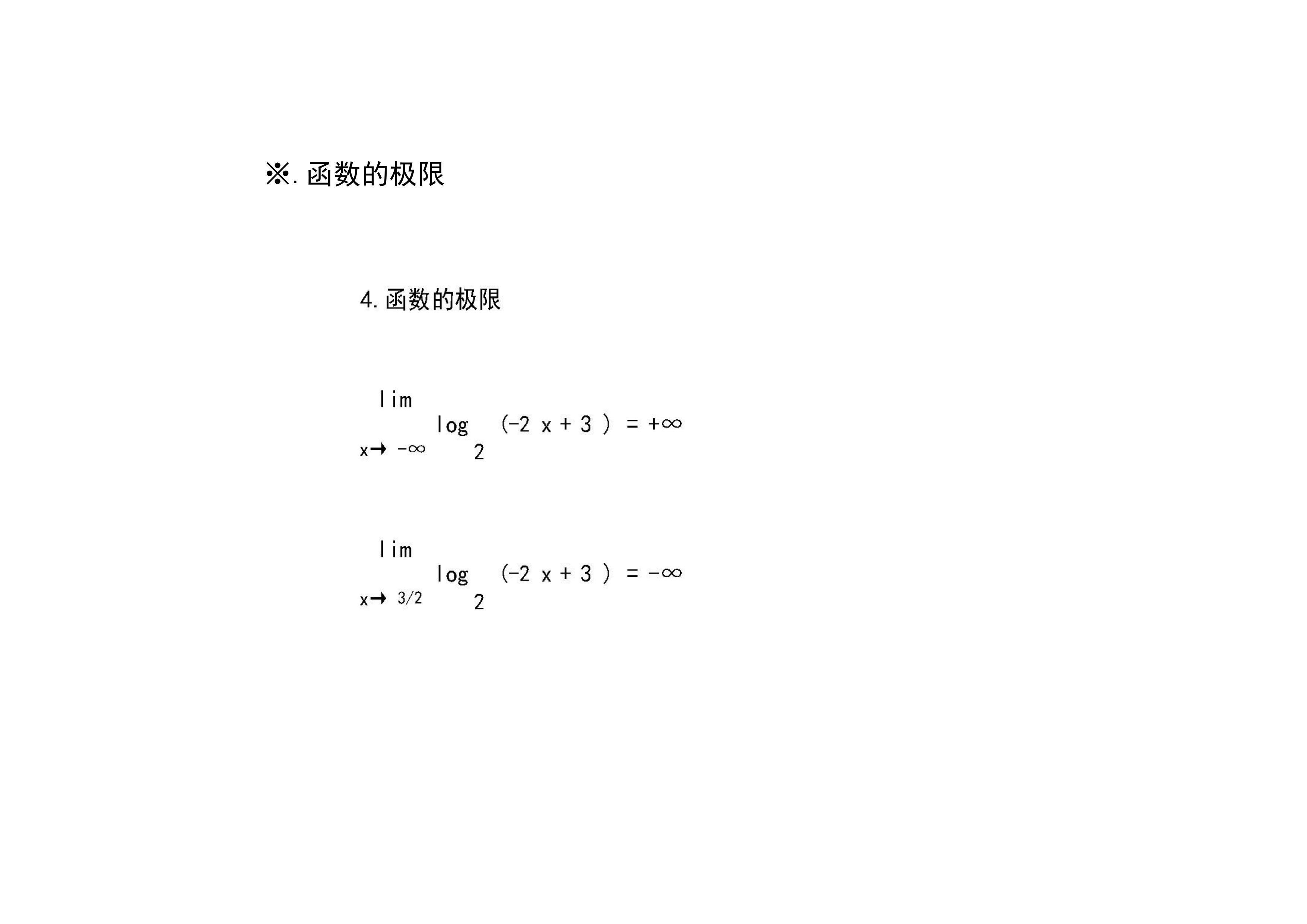
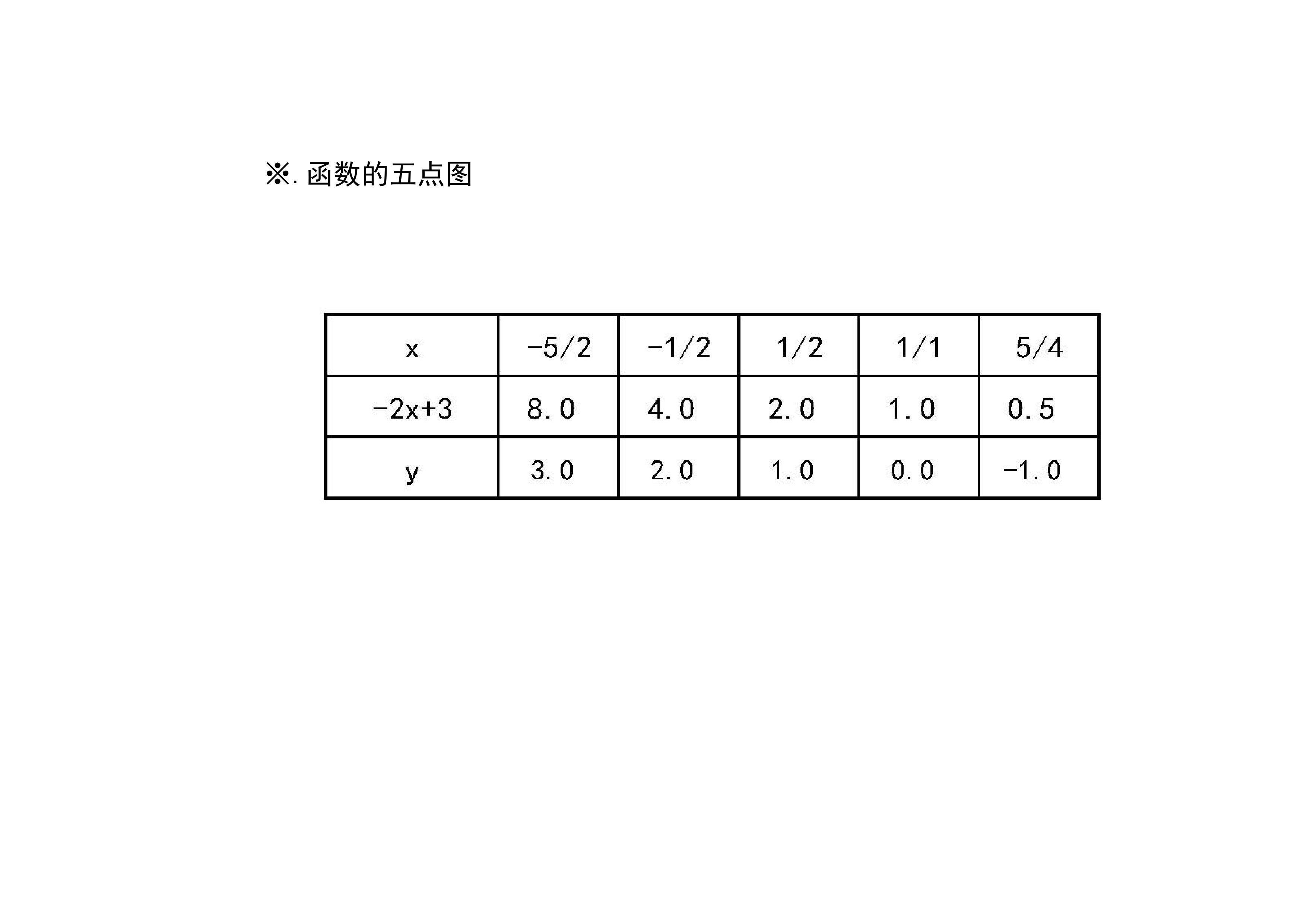
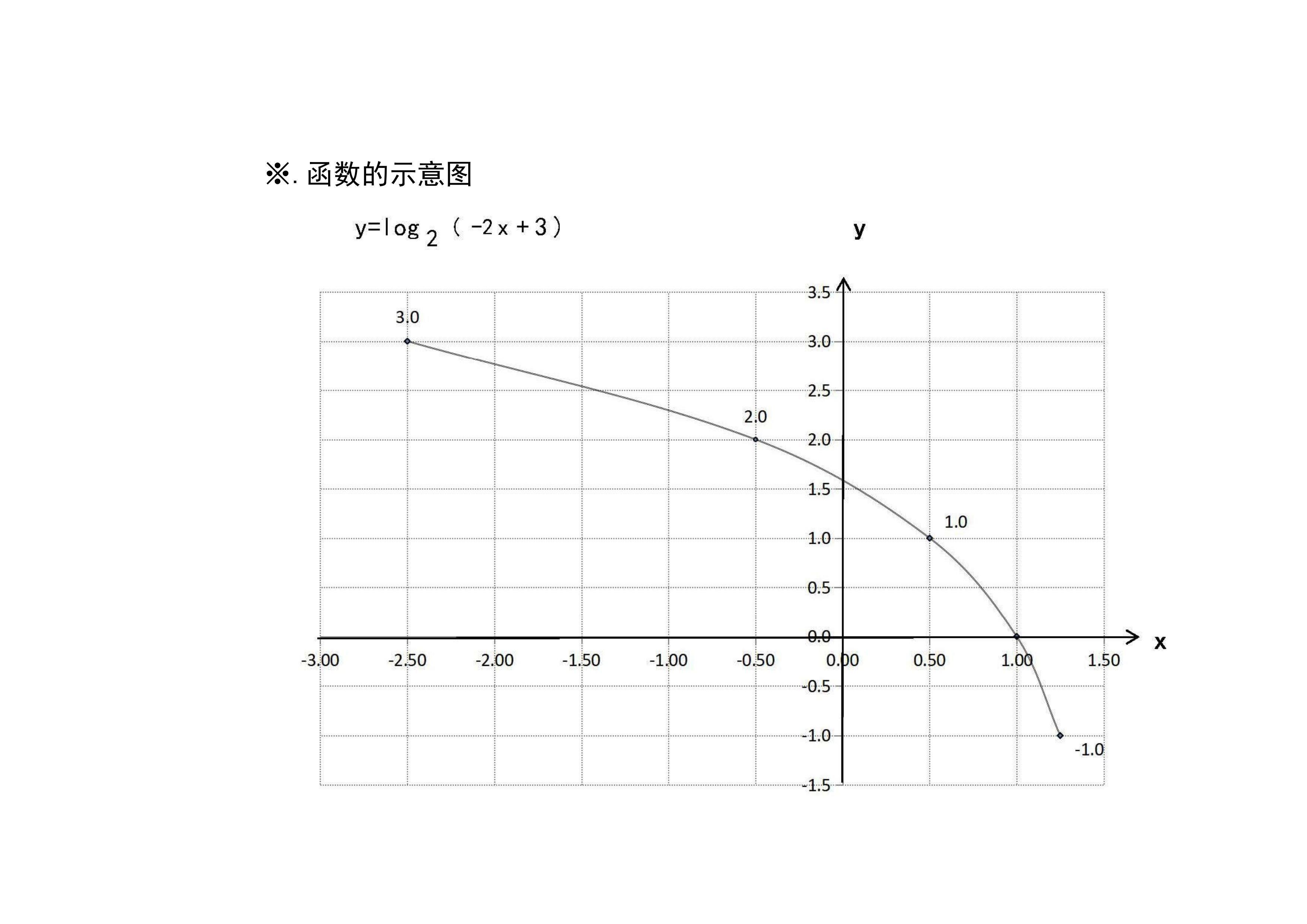
8.函数y=log2(-2x+3)的图像示意图：介绍函数的定义域、单调性、凸凹性、极限等性质，列举函数的五点图表，简要画出函数的示意图。

\n\n

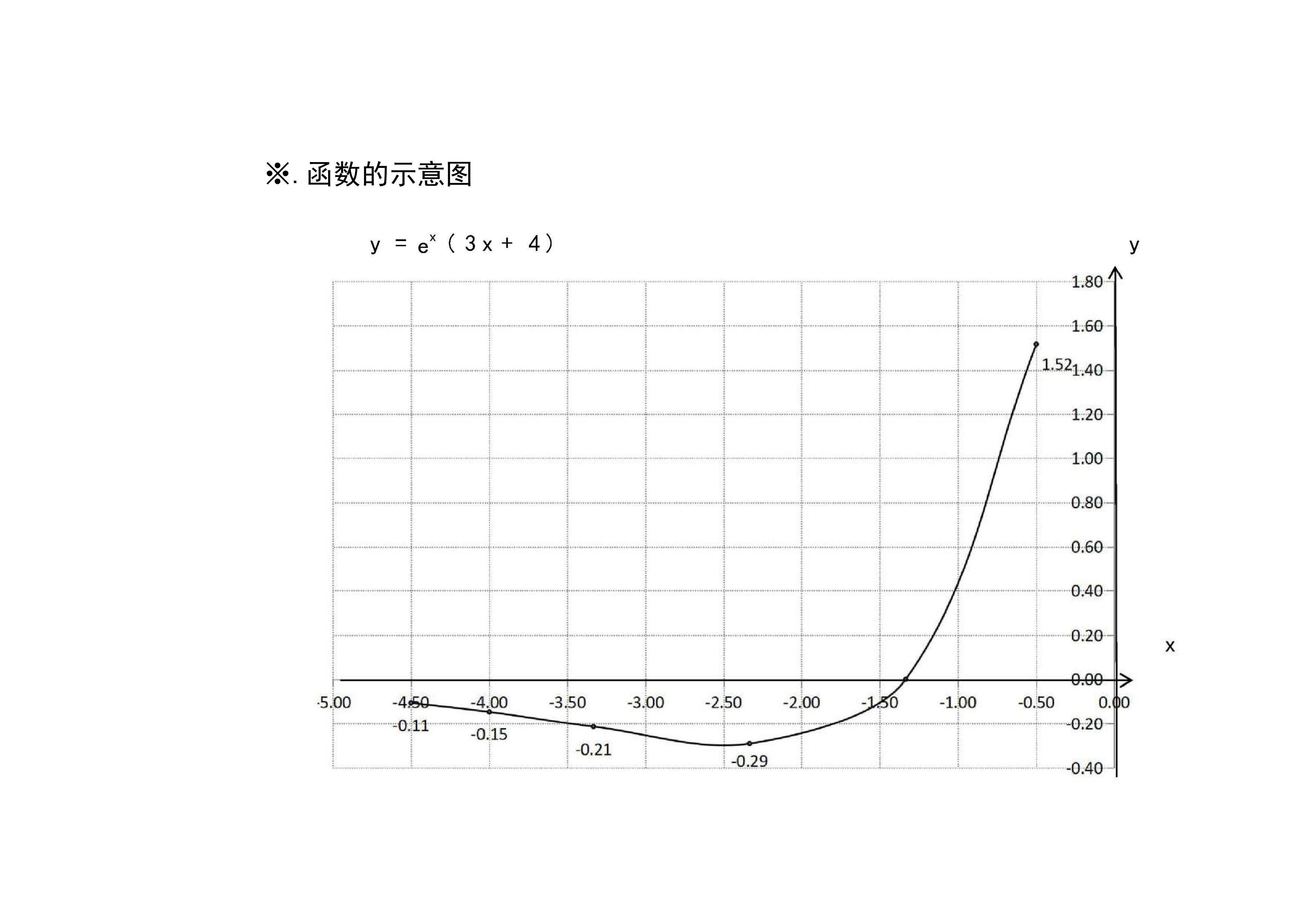
9.函数y=e^x(3x+4)的图像示意图：本文通过函数的定义、单调、凸凹性和极限等性质，介绍函数的主要性质及图像画法步骤。

\n\n

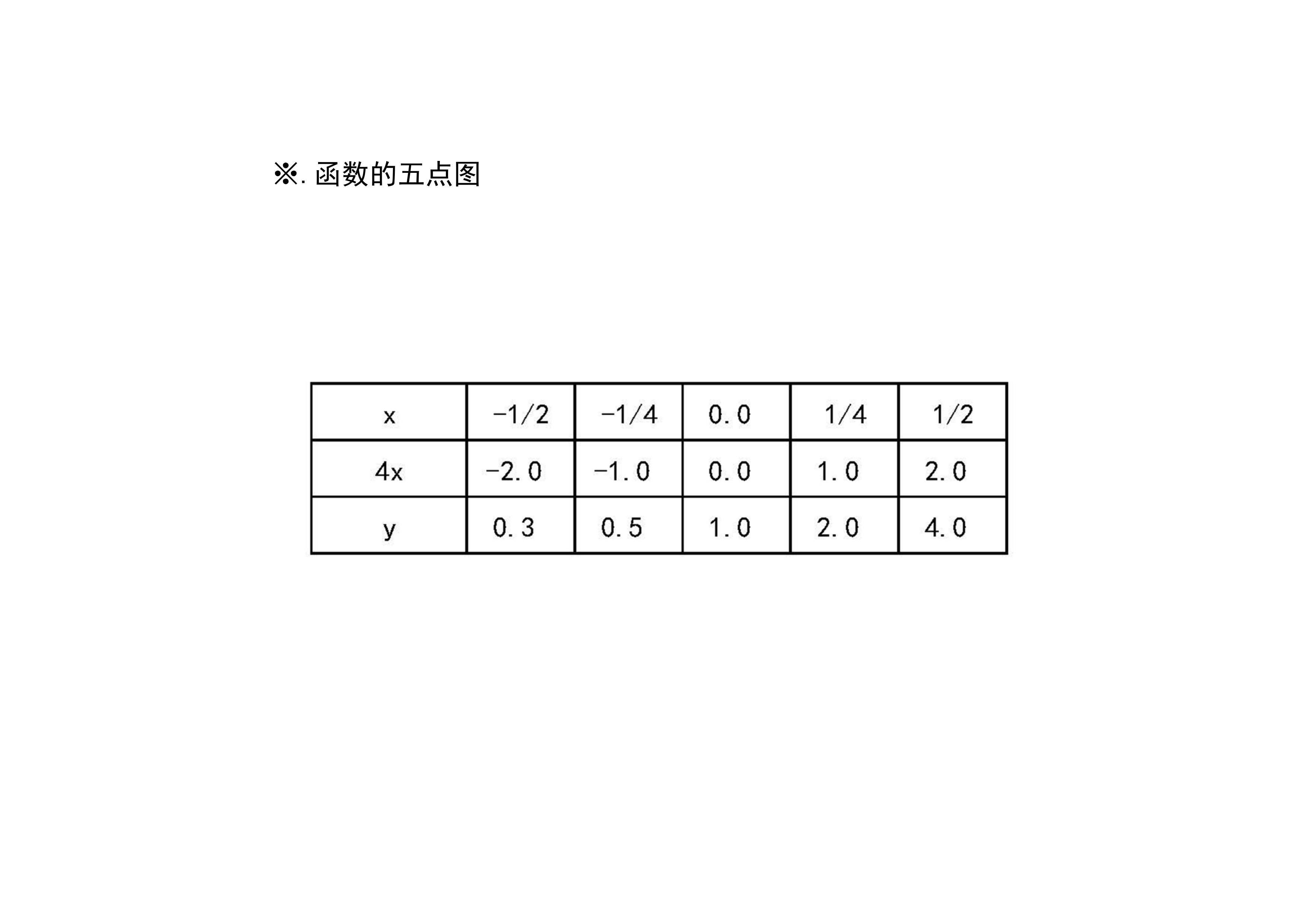
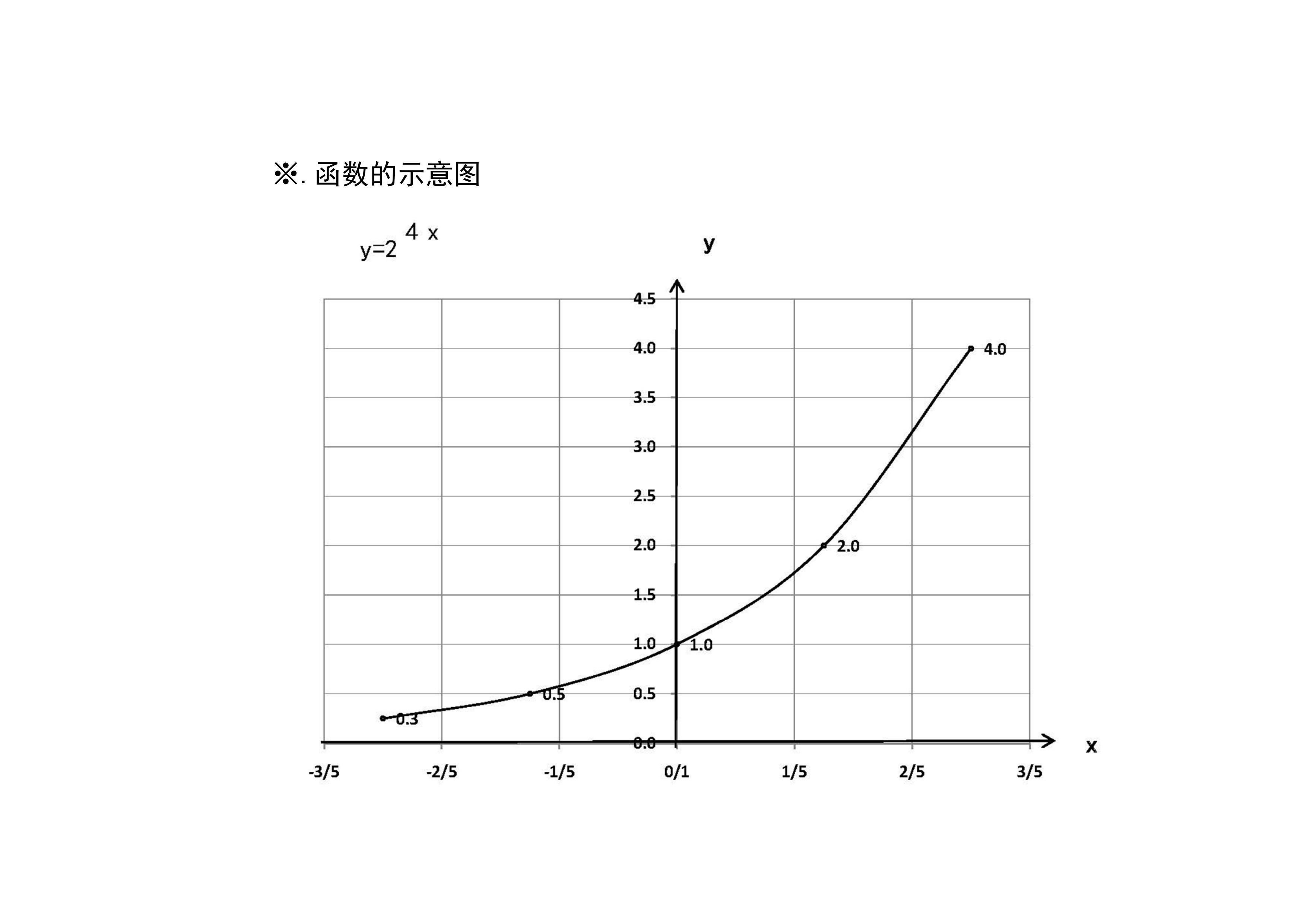
10.函数y=2^4x的图像示意图：介绍函数的定义域、单调性、凸凹性、极限等性质，列举函数的五点图表，进一步画出函数的示意图。

\n\n

\n\n

","gnid":"9758824ec7671aa4d","img_data":[{"flag":2,"img":[{"desc":"","height":925,"title":"","url":"https://p0.ssl.img.360kuai.com/t01a370aba0dc0184ae.jpg","width":1280},{"desc":"","height":925,"title":"","url":"https://p0.ssl.img.360kuai.com/t01fe729509bf6963a8.jpg","width":1280},{"desc":"","height":925,"title":"","url":"https://p0.ssl.img.360kuai.com/t01c644eeab94a0efe6.jpg","width":1280},{"desc":"","height":925,"title":"","url":"https://p0.ssl.img.360kuai.com/t013539215e0a83c2a4.jpg","width":1280},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t011ac510957e2c014e.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01b6300bde0321b12c.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t010ec62f9edc968eba.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t012ea8cadd7067c59a.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0135e6b9e5ad5f2723.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0101a95b931e2c6661.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0156869fd05f1b9bb9.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01eeb16f6ae9877b62.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01fd234f62dc83b806.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01a7177f0c14cf1b67.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01f3e9bcbfa66207be.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0111341fafd83e8b23.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01b12ae4529c0fc1a5.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01c2d414e70ce33022.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01a6435466e13d89c7.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0179a153481db89522.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t013f7979ab67311565.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t012871721b42a710c1.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t013b8fb94c7da143c9.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t015c6380a9e4dd5da4.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01c8edbb39b9d7fe87.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0122b1ab788e519a35.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01b446dfdddb35e4bf.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01651411583c303362.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0126094f1ca9ed1956.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t018fabf5214cdfc785.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t015b3f1e97b4dbe1ae.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01190830f3a32242fd.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01f1055028c526dd9d.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01e3ef63beab75c785.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t011dde9de6ce8a849b.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t019e8dbf2156e3031f.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t017d660aed63d492fa.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t017d660aed63d492fa.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0173f2a15f98505230.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0121e883d03506ad7f.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01fd20ffaf7942432d.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0174518a47ce9dd664.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t013e8e7b19fa695693.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0130fe2d9574be5db9.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0143c4b32aae3b438c.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01f74b752f697884fa.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0117c554a911c21419.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t0141cfbe536892d640.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01d396d571728906e5.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t01c87a38b737bd03fb.jpg","width":"3509"},{"desc":"","height":"2481","title":"","url":"https://p0.ssl.img.360kuai.com/t018eaaf25c81dbece5.jpg","width":"3509"}]}],"original":0,"pat":"pdc,art_src_0,fts0,sts0","powerby":"cache","pub_time":1711495200000,"pure":"","rawurl":"http://zm.news.so.com/7339c860ffebb01ebf215d79eb02f630","redirect":0,"rptid":"65e62da3ddba0208","rss_ext":[],"s":"t","src":"仁新数学","tag":[],"title":"导数五步法画函数图像10个函数示意图应用举例之一

宣汤宽2777曲线y=e^x/x,求水平渐近线和垂直渐近线. -
孔映仲15754788036 ______[答案] 可以看出曲线y=e^x/x的断点为x=0 当x→0+时,y→∞,故其垂直渐近线y=0 当x→-∞时,y→0,故其水平渐近线x=0

宣汤宽2777求曲线y=e^x的一条切线,使得该切线与曲线及直线x=0,x=2所围的平面图形的面积最小? -
孔映仲15754788036 ______[答案] 设点A(a,e^a)位于曲线上y=e^x的导数是y'=e^x,在A点的斜率为k=e^a那直线的方程可以写出来y-e^a=(e^a)(x-a),y=(e^a)(x-a+1)该切线与曲线及直线x=0,x=2所围的平面图形的面积为S=∫(0,2) (e^x)-(e^a)(x-a+1)dx=[(e^x)-(e...

宣汤宽2777求曲线y=e^x及该曲线过原点的切线与y轴所围成的平面图形的面积和该平面绕x轴旋转所得的体积.请问这道题如何做,这个图形怎么画? -
孔映仲15754788036 ______[答案] 1.求切线方程:设相切于(p,e^p),于是有切线方程:有y-e^p=e^p(x-p) 将原点代入有:-e^p=-pe^p,p=1 切线方程:y=ex 2.求所围面积:(1)曲线下面积:S1=∫[0,1]e^xdx=e^x|[0,1]=e-1 (2)三角形面积:S2=0.5*e*1^2=0.5e 所求面积:S=S1-S2=0.5e...

宣汤宽2777设曲线y=f(x)经过点(0,2),且曲线任一点(x,y)处的切线斜率为e^x,求此曲线的解析式答案上写的是:f(x)=e^x+1 -
孔映仲15754788036 ______[答案] 切线斜率就是y' 所以这里f'(x)=y'=e^x 则f(x)=∫e^xdx=e^x+C 且x=0,y=2 所以2=e^0+C C=1 所以f(x)=e^x+1

宣汤宽2777求曲线y=e^x,y=e^ - x及x=1所围成的图形.麻烦附图形,求平面图形的面积,不是画图! -
孔映仲15754788036 ______[答案] 由于 y = e^x 的图像在 y = e^(-x) 图像的上方, ∴ 所围面积 = ∫[e^x - e^(-x)]dx (x : 0 → 1) = ∫e^x dx + ∫ e^(-x)d(-x) (x : 0 → 1) = e^x + e^(-x) (x : 0 → 1) = e - 1 + e^(-1) - 1 = e + e^(-1) - 2 ≈ 1.08616

宣汤宽2777求由曲线y=e^x,y=e^ - x与直线x=1所围成立的平面形的面积.这样的题怎样去理解?积分上限与积分下限是如何算出的?谢谢! -
孔映仲15754788036 ______[答案] e^x=e^-x e^2x=1 2x=0 x=0 所以交点是(0,1) 所以积分上下限是1和0 0e^-x 所以面积是∫(0,1)(e^x-e^-x)dx =e^x+e^-x (0,1) =e+1/e-1-1 =e+1/e-2

宣汤宽2777y等于e的x次方图像是什么? -
孔映仲15754788036 ______ 函数 y = e^x 描述了以常数 e(自然对数的底数)为底的指数函数.它的图像是一条上升的曲线,以y轴为渐近线,永远不会与y轴相交.在x轴上,y = e^x 从左向右逐渐增加.具体来说,指数函数 e^x 在x = 0 处的值是 e^0 = 1,这是它的一个特殊...

宣汤宽2777一道高数题求由曲线Y=e^x.X轴及该曲线过原点的切线所围成图形的面积 -
孔映仲15754788036 ______[答案] 楼主题目错了 y=e^x是指数函数,不可能与x轴有交点的 更别说和它的切线与x轴能围成图形面积 题目应该改为Y=e^x.y轴及该曲线过原点的切线所围成图形的面积 具体做法请看图

宣汤宽2777曲线y=e^x - 1过点(0,0)处的切线方程 要原因 曲线y=e^x - 1过点(0,0)处的切线方程 -
孔映仲15754788036 ______[选项] A. y=x B. y=x-1 C. y=-x D. y=1-x

宣汤宽2777求曲线e^x+e^y=x+y+2在点P(0,0)处的切线 一阶导数不存在 -
孔映仲15754788036 ______[答案] 对e^x+e^y=x+y+2两边求导得 e^x+y'e^y=1+y' y'=(1-e^x)/(e^y-1) 显然当x=0,y=0时, y'=0/0型,所以y'(0)不存在